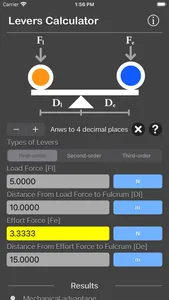
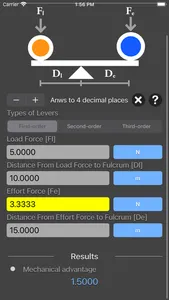
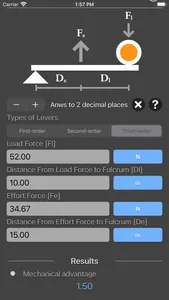
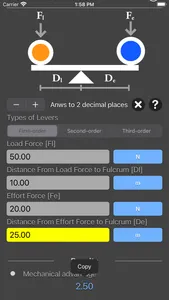
Levers Calculator are physic/math calculator to find effort force, load force, distance from load force to fulcrum, distance from effort force to fulcrum or Mechanical advantage fast and easy.
Features:
- Instant calculation
- Result are copy able to other app
- Formula are include as reference
- Support up to 16 decimal place
- Support various unit for each input
A lever is a simple machine consisting of a beam or rigid rod pivoted at a fixed hinge, or fulcrum. A lever is a rigid body capable of rotating on a point on itself. On the basis of the location of fulcrum, load and effort, the lever is divided into three types. It is one of the six simple machines identified by Renaissance scientists. A lever amplifies an input force to provide a greater output force, which is said to provide leverage. The ratio of the output force to the input force is the mechanical advantage of the lever.
Levers are classified by the relative positions of the fulcrum, effort and resistance (or load). It is common to call the input force the effort and the output force the load or the resistance. This allows the identification of three classes of levers by the relative locations of the fulcrum, the resistance and the effort:
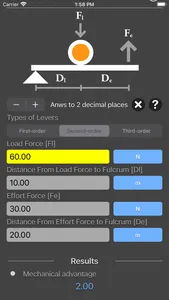
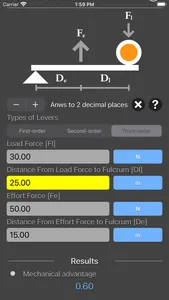
Class 1: Fulcrum in the middle: the effort is applied on one side of the fulcrum and the resistance (or load) on the other side, for example, a seesaw, a crowbar or a pair of scissors. Mechanical advantage may be greater than, less than, or equal to 1.
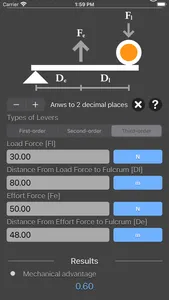
Class 2: Resistance (or load) in the middle: the effort is applied on one side of the resistance and the fulcrum is located on the other side, for example, a wheelbarrow, a nutcracker, a bottle opener or the brake pedal of a car. Load arm is smaller than the effort arm. Mechanical advantage is always greater than 1. It is also called force multiplier lever.
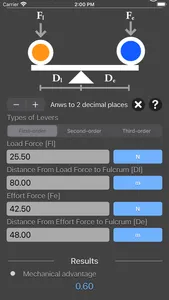
Class 3: Effort in the middle: the resistance (or load) is on one side of the effort and the fulcrum is located on the other side, for example, a pair of tweezers or the human mandible. The effort arm is smaller than the load arm. Mechanical advantage is always less than 1. It is also called speed multiplier lever.
Mechanical advantage is a measure of the force amplification achieved by using a tool, mechanical device or machine system. The device preserves the input power and simply trades off forces against movement to obtain a desired amplification in the output force. The model for this is the law of the lever. Machine components designed to manage forces and movement in this way are called mechanisms. An ideal mechanism transmits power without adding to or subtracting from it. This means the ideal mechanism does not include a power source, is friction less, and is constructed from rigid bodies that do not deflect or wear. The performance of a real system relative to this ideal is expressed in terms of efficiency factors that take into account departures from the ideal.
The moment action on both sides of the lever is equal and can be expressed as
Fe x De = Fl x Dl
where
Fe = effort force (N, lb)
Fl = load force (N, lb) (note that weight is a force)
Dl = distance from load force to fulcrum (m, ft)
De = distance from effort force to fulcrum (m, ft)
The effort force can be calculated by modifying to
Fe = Fl x Dl / De
Fe = m x g x Dl / De
where
m = mass (kg)
g = acceleration of gravity (9.81 m/s²)
Thanks for your support and do visit nitrio.com for more apps for your iOS devices.
Features:
- Instant calculation
- Result are copy able to other app
- Formula are include as reference
- Support up to 16 decimal place
- Support various unit for each input
A lever is a simple machine consisting of a beam or rigid rod pivoted at a fixed hinge, or fulcrum. A lever is a rigid body capable of rotating on a point on itself. On the basis of the location of fulcrum, load and effort, the lever is divided into three types. It is one of the six simple machines identified by Renaissance scientists. A lever amplifies an input force to provide a greater output force, which is said to provide leverage. The ratio of the output force to the input force is the mechanical advantage of the lever.
Levers are classified by the relative positions of the fulcrum, effort and resistance (or load). It is common to call the input force the effort and the output force the load or the resistance. This allows the identification of three classes of levers by the relative locations of the fulcrum, the resistance and the effort:
Class 1: Fulcrum in the middle: the effort is applied on one side of the fulcrum and the resistance (or load) on the other side, for example, a seesaw, a crowbar or a pair of scissors. Mechanical advantage may be greater than, less than, or equal to 1.
Class 2: Resistance (or load) in the middle: the effort is applied on one side of the resistance and the fulcrum is located on the other side, for example, a wheelbarrow, a nutcracker, a bottle opener or the brake pedal of a car. Load arm is smaller than the effort arm. Mechanical advantage is always greater than 1. It is also called force multiplier lever.
Class 3: Effort in the middle: the resistance (or load) is on one side of the effort and the fulcrum is located on the other side, for example, a pair of tweezers or the human mandible. The effort arm is smaller than the load arm. Mechanical advantage is always less than 1. It is also called speed multiplier lever.
Mechanical advantage is a measure of the force amplification achieved by using a tool, mechanical device or machine system. The device preserves the input power and simply trades off forces against movement to obtain a desired amplification in the output force. The model for this is the law of the lever. Machine components designed to manage forces and movement in this way are called mechanisms. An ideal mechanism transmits power without adding to or subtracting from it. This means the ideal mechanism does not include a power source, is friction less, and is constructed from rigid bodies that do not deflect or wear. The performance of a real system relative to this ideal is expressed in terms of efficiency factors that take into account departures from the ideal.
The moment action on both sides of the lever is equal and can be expressed as
Fe x De = Fl x Dl
where
Fe = effort force (N, lb)
Fl = load force (N, lb) (note that weight is a force)
Dl = distance from load force to fulcrum (m, ft)
De = distance from effort force to fulcrum (m, ft)
The effort force can be calculated by modifying to
Fe = Fl x Dl / De
Fe = m x g x Dl / De
where
m = mass (kg)
g = acceleration of gravity (9.81 m/s²)
Thanks for your support and do visit nitrio.com for more apps for your iOS devices.
Show More